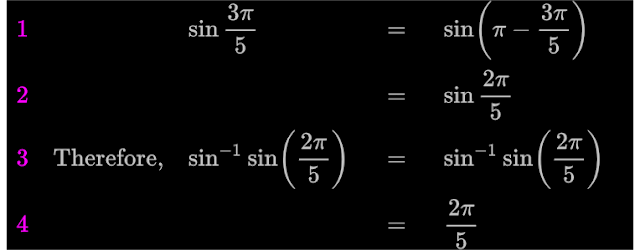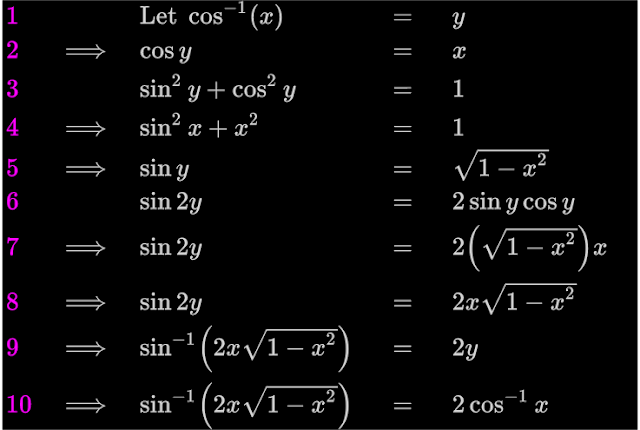In the previous section,
we saw tan-1 function. In
this section, we will see cot-1 function.
Some basics can be written in 8 steps:
1. Consider the cot function:
f(x) = cot x
◼ For this function, we must choose the input values carefully. It can be written in 3 steps:
(i) We know that: $\cot x = \frac{\cos x}{\sin x}$.
• The denominator should not become zero. That means, sin x should not become zero.
(ii) We know that:
♦ sin 0 = 0
♦ sin π = 0
♦ sin (-π) = 0
♦ sin 2π = 0
♦ sin (-2π) = 0
♦ sin 3π = 0
♦ so on . . .
• So we can write:
The input x should not be equal to nπ, where n is an integer.
(iii) Thus we get the domain for f(x) = cot x as:
set {x : x∈R and x ≠ nπ, n∈Z}
• That means, x can be any real number except nπ, where n is an integer.
◼ Similarly, we must have a good knowledge about the codomain of cot x. It can be written in 3 steps:
(i) We know that, output of sin x will lie in the interval [-1,1].
• That means, output of sin x will be any one of the four items below:
♦ -1
♦ a -ve proper fraction
♦ a -ve proper fraction
♦ +1
(Though
zero lies in the interval [-1,1], we are not allowing sin x to become
zero. We achieve this by avoiding nπ as input x values)
(ii) Based on the above "possible outputs of sin x", we can write the "possible outputs of cot x":
• Remember that, the numerator cos x can give any output in the interval [-1,1].
So the output of $\frac{\cos x}{\sin x}$ can be any real number.
For example:
$\cot x = \frac{\cos x}{\sin x} = \frac{\frac{7}{67}}{\frac{98}{99}} ~=~ 0.1051$
(iii) Thus we get the codomain of cot x as: R
• We saw the above details in class 11. We saw a neat pictorial representation of the above details in the graph of the cot function. It is shown again in fig.18.22 below:
 |
| Fig.18.22 |
2. Let us check whether the cot function is one-one.
• Let input x = $\frac{\pi}{2}$.
♦ Then the output will be $f \left(\frac{\pi}{2} \right)~=~\cot \left(\frac{\pi}{2} \right)~=~0 $
• Let input x = $\frac{- \pi}{2}$.
♦ Then the output will be $f \left(\frac{- \pi}{2} \right)~=~\cot \left(\frac{- \pi}{2} \right)~=~0 $
• Let input x = $\frac{-3 \pi}{2}$.
♦ Then the output will be $f \left(\frac{-3 \pi}{2} \right)~=~\cot \left(\frac{-3 \pi}{2} \right)~=~0 $
(We can cross check with the graph and confirm that the above inputs and outputs are correct)
•
We see that, more than one input values from the domain can give the
same output. So the cot function is not a one-one function.
3. Suppose that, we restrict the input values.
• That is, we take input values only from the set $\left(0, \pi \right)$.
♦ Note that, we use '()' instead of '[]'.
♦ That means, the boundary values should not be used as inputs.
• We already saw the codomain. It is: R
• Then we will get the green curve shown in fig.18.23 below:
 |
| Fig.18.23 |
• In the green portion, no two inputs will give the same output. So the green portion represents a function which is one-one.
4. Next, we have to prove that, the green portion is onto.
• For that, we can consider any y value from the codomain R.
•
There will be always a x value in $\left(0, \pi \right)$, which will satisfy the equation y = cot x.
• So the green portion is onto.
5. We see that, the green portion is both one-one and onto.
• We can represent this function in the mathematical way:
$\text{cot}:~ \left(0, \pi \right)~\to~R$, defined as f(x) = cot x.
6. If a function is both one-one and onto, the codomain is same as range.
• So we can write:
For this function,
♦ the domain is $\left(0, \pi \right)$
♦ the range is R
7.
We know that, if a function is one-one and onto, it will be invertible.
We have seen the properties of inverse functions. Let us apply those
properties to our present case. It can be written in 4 steps:
(i) If y = f(x) = cot x is invertible, then there exists a function g such that: g(y) = x
(ii) The function g will also be one-one and onto.
(iii) The domain of f will be the range of g. So the range of g is $\left(0, \pi \right)$.
(iv) The range of f will be the domain of g. So the domain of g is R
(iv) The inverse of cot function is denoted as cot-1. So we can define the inverse function as:
$\cot^{-1}:~ R~\to~\left(0, \pi \right)$, defined as x = g(y) = cot-1 y.
8. Let us see an example:
• Suppose that, for the inverse function, the input y is $\sqrt{3}$
• Then we get an equation: $x ~=~\cot^{-1} \left(\sqrt{3} \right)$
• Our aim is to find x. It can be done in 4 steps:
(i) $x ~=~\cot^{-1} \left(\sqrt{3} \right)$ is an equation of the form $x ~=~f(y)~=~\cot^{-1} \left(y \right)$
(ii) This is an inverse trigonometric function, where input y = $\sqrt{3}$.
•
Based on the inverse trigonometric function, we can write the original trigonometric function:
$y ~=~ f(x) ~=~\cot x$
•
In our present case, it is:
$y~=~\sqrt{3}~=~\cot x$
(iii) So we have a trigonometric equation:
$\cot x~=~\sqrt{3}$
•
When we solve this equation, we get x.
(iv) We have seen the method for solving trigonometric equations in class 11.
•
In the present case, we do not need to write many steps. We already know that, $\cot \left(\frac{\pi}{6} \right)~=~\sqrt{3}$
•
So we can write:
$x ~=~\frac{\pi}{6}$
The
above 8 steps help us to understand the basics about cosec-1 function. Now we will see a few more details. It can be
written in 4 steps:
1. We saw that, the cot function is not a one-one
function. But to make it one-one, we restricted the domain to $\left(0, \pi \right)$.
2. There are other possible “restricted domains” available.
• $\left(- \pi, 0 \right)$ is shown in magenta color in fig.18.24 below:
 |
| Fig.18.24 |
• $\left(-2 \pi, -\pi \right)$ is shown in cyan color in fig,18.12 above.
3. There are infinite number of such restricted domains possible.
• We say that:
♦ Each restricted domain gives a corresponding
branch of the cot
-1 function.
♦ The restricted domain $\left(0, \pi \right)$ gives the
principal branch of the cot
-1 function.
4. In class 11, we plotted the cot function. Now we will plot the inverse. It can be done in 4 steps:
(i) Write the set for the original function f. It must contain a convenient number of ordered pairs.
• $\left(\frac{\pi}{6} , \sqrt{3} \right)$ is an example of the ordered pairs in f.
(To get a smooth curve, we must write a large number of ordered pairs)
(ii) Based on set f, we can write set g.
This is done by picking each ordered pair from f and interchanging the positions.
•
For example, the point $\left(\frac{\pi}{6} , \sqrt{3} \right)$ in
the set f will become $\left(\sqrt{3} , \frac{\pi}{6} \right)$ in
set g.
• Thus we will get the required number of ordered pairs in g.
(iii) Mark each ordered pair of g on the graph paper.
• The first coordinate should be marked along the x-axis.
• The second coordinate should be marked along the y-axis.
(iv) Once all the ordered pairs are marked, draw a smooth curve connecting all the marks.
• The smooth curve is the required graph. It is shown in fig.18.25 below:
 |
| Fig.18.25 |
(v) Unlike the graphs of sin-1 and cos-1, the graph of cosec-1 is not smaller in width. This is because:
Any
real number, can be used as input
values. That means, the graph can extend upto -∞ towards the left and
upto +∞ towards the right.
• The graph is larger in height because:
♦ Depending upon the branch, values upto +∞ or –∞ can be obtained as output values.
• The green curve is related to the $\left(0, \pi \right)$ branch.
• The cyan curve is related to the $\left(\pi, 2 \pi \right)$ branch.
• The magenta curve is related to the $\left(- \pi, 0 \right)$ branch.
We have seen all the six inverse trigonometric functions. In the next section, we will see some solved examples.
Previous
Contents
Next
Copyright©2024 Higher secondary mathematics.blogspot.com